Intro
Discover the square root of 36, a fundamental math concept, and learn how to calculate it using simple arithmetic operations and radical expressions, understanding perfect squares and roots.
The square root of a number is a value that, when multiplied by itself, gives the original number. In this case, we are looking for the square root of 36. To find the square root of 36, we need to determine what number, when multiplied by itself, equals 36.
The square root of 36 is 6, because 6 multiplied by 6 equals 36. This can be expressed mathematically as √36 = 6, where the symbol √ represents the square root. The square root of a number is a fundamental concept in mathematics and is used extensively in various mathematical operations, including algebra, geometry, and calculus.
In simple terms, the square root of 36 is the number that, when squared (multiplied by itself), gives 36. Since 6 * 6 = 36, the square root of 36 is 6. Understanding square roots is important for solving equations and working with mathematical formulas that involve squared numbers or variables.
Introduction to Square Roots

Square roots are used to find the length of a side of a square when we know the area. For example, if a square has an area of 36 square units, the length of each side would be the square root of 36, which is 6 units.
How to Calculate Square Roots
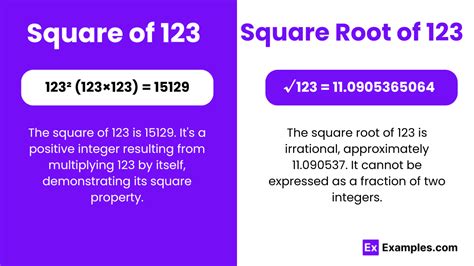
Calculating square roots can be done using various methods, including long division, factoring, or using a calculator. For simple numbers like 36, we can easily find the square root by recognizing perfect squares. However, for more complex numbers, we may need to use a calculator or a mathematical algorithm.
Importance of Square Roots in Mathematics
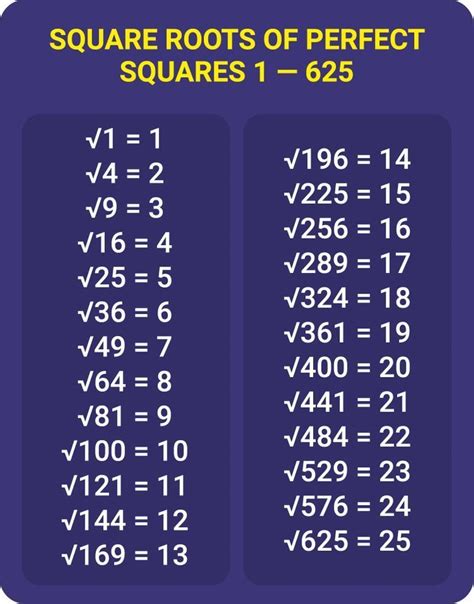
Square roots play a crucial role in mathematics, particularly in algebra and geometry. They help in solving quadratic equations, finding distances and lengths in geometric shapes, and are essential in various mathematical formulas and theorems.
Real-World Applications of Square Roots
Beyond mathematics, square roots have numerous real-world applications. They are used in physics to calculate distances and speeds, in engineering to design structures and machines, and in finance to model economic trends and calculate returns on investments.
Challenges in Working with Square Roots

Working with square roots can sometimes be challenging, especially when dealing with irrational numbers or complex equations. However, understanding the principles and practicing calculations can help overcome these challenges.
Tools and Resources for Square Root Calculations

There are various tools and resources available for calculating square roots, including calculators, online square root calculators, and mathematical software. These tools can simplify the process of finding square roots and make it more efficient.
Conclusion and Future Directions

In conclusion, understanding square roots is essential for advancing in mathematics and applying mathematical concepts to real-world problems. As mathematics continues to evolve, the importance of square roots will remain, and their applications will expand into new areas.
Gallery of Square Root Images
Square Root Image Gallery
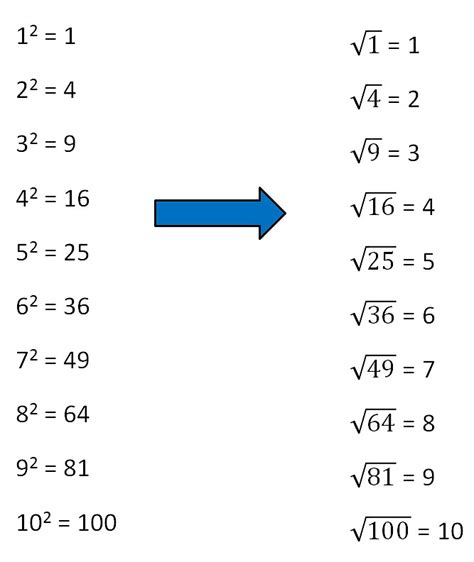





Frequently Asked Questions
What is the square root of 36?
+The square root of 36 is 6, because 6 multiplied by 6 equals 36.
How do you calculate square roots?
+Calculating square roots can be done using various methods, including long division, factoring, or using a calculator.
What are the real-world applications of square roots?
+Square roots have numerous real-world applications, including physics, engineering, finance, and more, where they are used to calculate distances, speeds, and returns on investments.
To further explore the concept of square roots and their applications, we invite readers to share their thoughts and experiences in the comments below. Whether you're a student looking to deepen your understanding of mathematical concepts or a professional seeking to apply square roots in your field, your insights are valuable. Share this article with others who might benefit from learning about square roots, and let's continue the conversation on the importance and versatility of square roots in mathematics and beyond.
